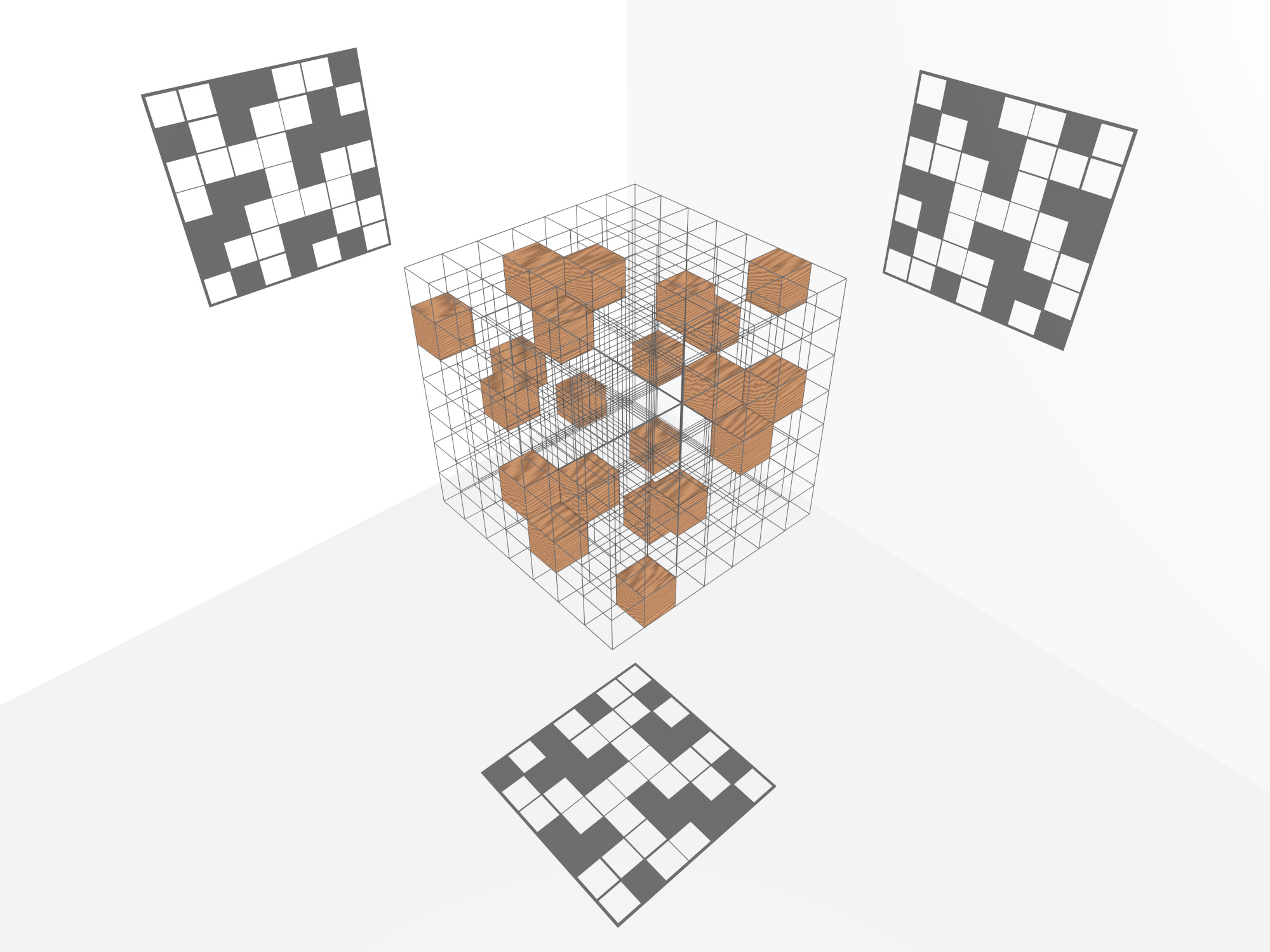
Projection cubes of symmetric designs

Projection cubes of symmetric designs were introduced in the paper [5]. A similar concept was earlier studied in [4]. A (v,k,λ) projection n-cube is an n-dimensional matrix with {0,1}-entries such that every 2-dimensional projection is an incidence matrix of a symmetric (v,k,λ) design. The set of all such objects is denoted Pⁿ(v,k,λ). Here are pictures of three P³(7,3,1)-cubes rendered in POV-Ray:

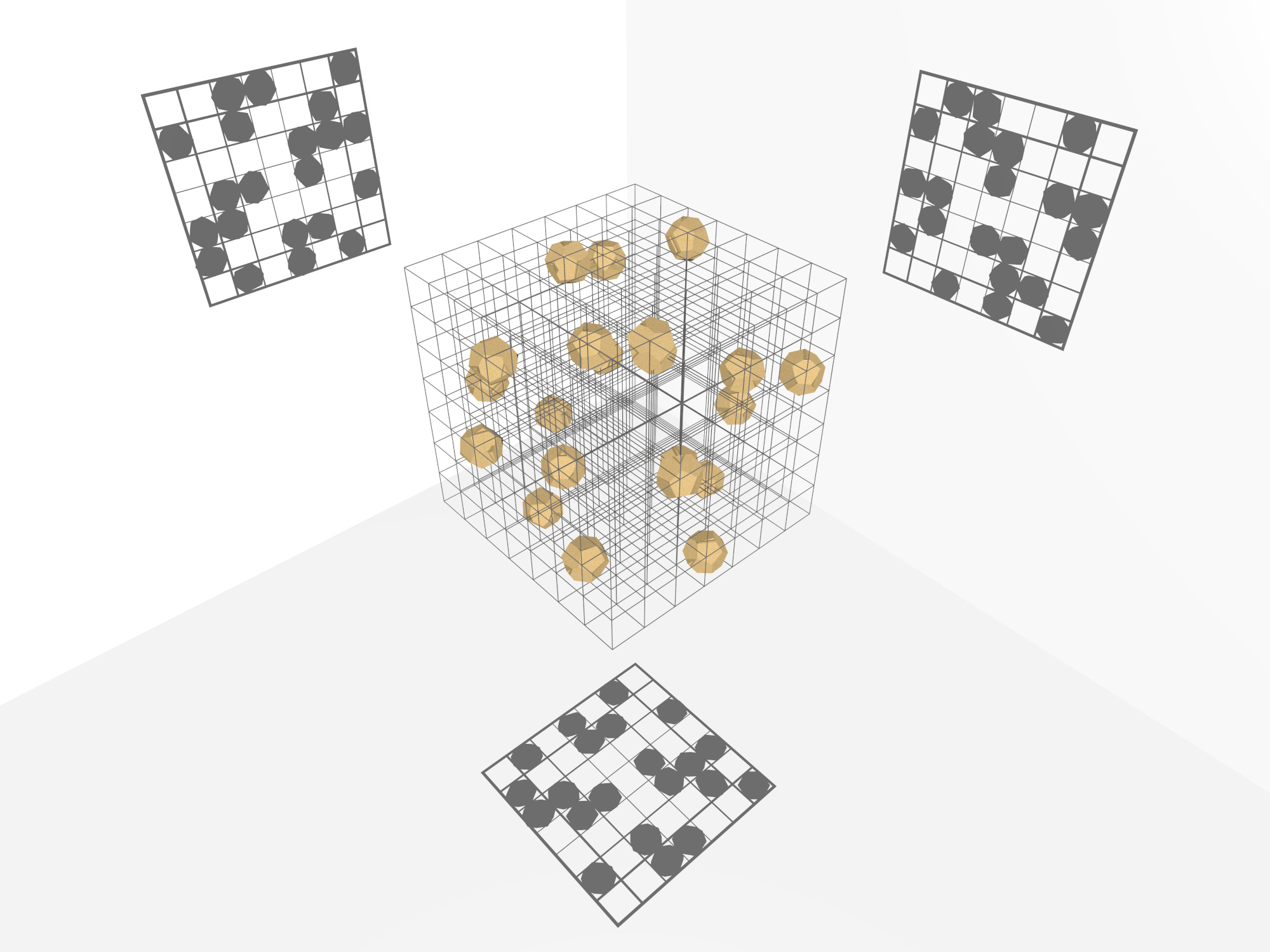
These are the cubes C₁, C₂, and C₃ from [5]. The file examples.oa contains all examples from the paper in GAP-compatible format. They can be explored using commands from the PAG package. Instructions and examples are available in the PAG manual. The following table contains lower bounds on the number of inequivalent cubes in Pⁿ(v,k,λ) with link to files in GAP format. These examples were constructed using n-dimensional difference sets, see [5].

[5], TABLE 4. Lower bounds on the number of Pⁿ(v,k,λ)-cubes.

There are at least 102 inequivalent projection cubes in P³(16,6,2) that cannot be obtained from difference sets. They were constructed by prescribing autotopy groups and using a Kramer-Mesner-like procedure [5]. A GAP file with these examples is available here.

More results about projection cubes were obtained in the follow up paper [6]. A complete classification of Pⁿ(7,3,1) and Pⁿ(7,4,2)-cubes was performed. All examples up to equivalence are given in the next table, along with the smallest nontrivial cubes Pⁿ(3,2,1).

[6], TABLE 1. Numbers of Pⁿ(v,k,λ)-cubes up to equivalence.

For the next parameters (11,5,2) we could not perform complete classification, but we found all Pⁿ(11,5,2)-cubes with nontrivial autotopies. A new algorithm for constructing P-cubes with prescribed autotopies by successively increasing the dimension was used.

[6], TABLE 2. The Pⁿ(11,5,2)-cubes with nontrivial autotopies.

Using the algorithm we could also find all P³(16,6,2)-cubes with an autotopy of order 8 acting semiregularly. There are 1076 such cubes in total. The following table gives their distribution by the projections and full auto(para)topy group sizes. There are three (16,6,2) designs up to isomorphism called the red, green, and blue design in [5, 6] and denoted by R, G, and B in the table. All possible combinations (with repetition) of three designs occur as projections. The group sizes are denoted by T=|Atop(C)| and P=|Apar(C)|/|Atop(C)|.

[6], TABLE 3. P³(16,6,2)-cubes with an autotopy of order 8 acting semiregularly.

In [5], a question was posed whether there exist P³(16,6,2)-cubes with three pairwise non-isomorphic (16,6,2) designs appearing as projections. From the table, we see that there are 152 such cubes with the prescribed autotopy of order 8. A picture of one of them was rendered in POV-Ray with red, green, and blue light sources placed so that the projections appear as shadows in the appropriate color:

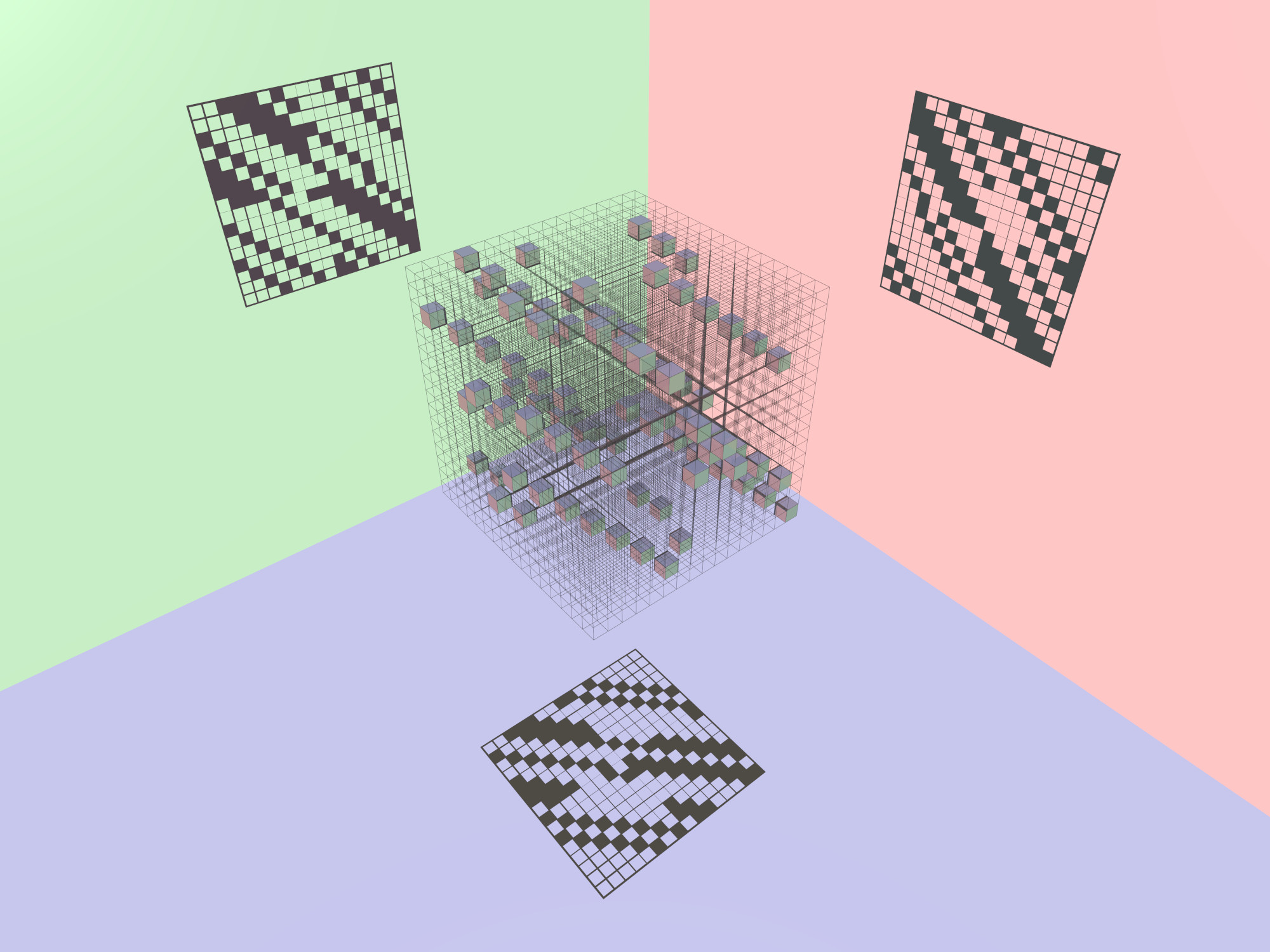
The final table in [6] contains numbers of inequivalent P-cubes constructed from n-dimensional difference sets in small groups G. A classification of n-dimensional difference sets was performed using an algorithm implemented in the programming language C. Several theorems and conjectures from the paper are based on this data.

[6], TABLE 4. Numbers of inequivalent Pⁿ(v,k,λ)-cubes obtained from difference sets.

References

1. The GAP Group, GAP – Groups, Algorithms, and Programming, Version 4.13.1, 2024. https://www.gap-system.org
2. V. Krcadinac, Prescribed Automorphism Groups, Version 0.2.4, 2024 (GAP package). https://vkrcadinac.github.io/PAG
3. V. Krcadinac, The PAG manual, 2024. https://web.math.pmf.unizg.hr/acco/PAGmanual.pdf
4. V. Krcadinac, M. O. Pavcevic, K. Tabak, Cubes of symmetric designs, Ars Math. Contemp. (2024). https://doi.org/10.26493/1855-3974.3222.e53
5. V. Krcadinac, L. Relic, Projection cubes of symmetric designs, preprint, 2024. https://arxiv.org/abs/2411.06936
6. V. Krcadinac, M. O. Pavcevic, On higher-dimensional symmetric designs, preprint, 2024. https://arxiv.org/abs/2412.09067
7. Persistence of Vision Raytracer, Version 3.7, 2013. http://www.povray.org

Vedran Krcadinac,