Glasnik Matematicki, Vol. 44, No.2 (2009), 333-342.
ON CERTAIN CHARACTER SUMS OVER SMOOTH
NUMBERS
Ke Gong
Department of Mathematics, Tongji University, Shanghai 200092, P. R. China
e-mail: kogong@gmail.com
Abstract. We give nontrivial bounds in various ranges for character sums of the form
∑n 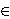 S(x,y)
χ(R1(n))eq(R2(n)),
S(x,y)
χ(R1(n))eq(R2(n)),
where χ is a nonprincipal multiplicative character modulo a prime q,
R1 and R2 are rational functions
modulo q, and S(x,y)
is the set of positive integers
n ≤ x that are divisible only by primes
p ≤ y.
We also give sharper bounds in some special cases.
2000 Mathematics Subject Classification.
11L40, 11L20, 11N25.
Key words and phrases. Character sums, rational functions, smooth numbers.
Full text (PDF) (free access)
DOI: 10.3336/gm.44.2.06
References:
- J. Bourgain,
More on the sum-product phenomenon in prime fields and its applications,
Int. J. Number Theory 1 (2005), 1-32.
MathSciNet
CrossRef
- R. de la Bretèche, G. Tenenbaum,
Sommes d'exponentielles friables d'arguments rationnels,
Funct. Approx. Comment. Math. 37 (2007), 31-38.
MathSciNet
- É. Fouvry, P. Michel,
Sur certaines sommes d'exponentielles sur les nombres premiers,
Ann. Sci. École Norm. Sup. (4) 31 (1998), 93-130.
MathSciNet
CrossRef
- É. Fouvry, G. Tenenbaum,
Entiers sans grand facteur premier en progressions arithmetiques,
Proc. London Math. Soc. (3) 63 (1991), 449-494.
MathSciNet
CrossRef
- H. Iwaniec, E. Kowalski,
Analytic Number Theory, American Mathematical Society, Providence, RI, 2004.
MathSciNet
- A. A. Karatsuba,
Sums of characters with prime numbers, Math. USSR Izv. 4 (1970), 303-326.
CrossRef
- A. A. Karatsuba,
Sums of Legendre symbols of polynomials of second degree over prime numbers,
Math. USSR Izv. 12 (1978), 299-308.
CrossRef
- A. A. Karatsuba,
Arithmetic problems in the theory of Dirichlet characters,
Russ. Math. Surv. 63 (2008), 641-690.
MathSciNet
CrossRef
- A. A. Kopaneva,
Application of Postnikov's formula for estimation of short sums of
Dirichlet characters over shifted prime numbers, Moscow Univ. Math. Bull. 63 (2008), 218-220.
CrossRef
- H. Maier,
Exponential sums with multiplicative coefficients over smooth integers,
Funct. Approx. Comment. Math. 35 (2006), 209-218.
MathSciNet
- G. I. Perel'muter,
On some sums with characters, Uspekhi Mat. Nauk 18 (1963), 145-149. (Russian)
- G. I. Perel'muter,
Evaluation of a sum containing primes, Soviet Math. Dokl. 3 (1962), 663-667.
- Z. Kh. Rakhmonov,
On the distribution of values of Dirichlet characters and their applications,
Proc. Steklov Inst. Math. 207 (1995), 263-272.
MathSciNet
- I. E. Shparlinski,
Character sums over shifted smooth numbers,
Proc. Amer. Math. Soc. 135 (2007), 2699-2705.
MathSciNet
CrossRef
- R. C. Vaughan,
A new iterative method in Waring's problem,
Acta Math. 162 (1989), 1-71.
MathSciNet
CrossRef
Glasnik Matematicki Home Page
![]() S(x,y)
χ(R1(n))eq(R2(n)),
S(x,y)
χ(R1(n))eq(R2(n)),