Glasnik Matematicki, Vol. 42, No.1 (2007), 69-82.

HOMOTOPY CHARACTERIZATION OF G-ANR'S

Natella Antonyan, Sergey A. Antonyan and Alejandra Soria-Pérez

Departamento de Matemáticas, Facultad de Ciencias, Universidad Nacional Autónoma de México, 04510 México Distrito Federal, México
e-mail: antonyan@servidor.unam.mx

Escuela de Matemáticas, Universidad Juárez del Estado de Durango, 34120 Durango, Dgo., México
e-mail: ale_godel@hotmail.com

Abstract.   Let G be a compact Lie group. We prove that if each point x 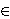X of a G-space X admits a G_x-invariant neighborhood U which is a G_x-ANE then X is a G-ANE, where G_x stands for the stabilizer of x. This result is further applied to give two equivariant homotopy characterizations of G-ANR's. One of them sounds as follows: a metrizable G-space Y is a G-ANR iff Y is locally G-contractible and every metrizable closed G-pair (X, A) has the G-equivariant homotopy extension property with respect to Y. In the same terms we also characterize G-ANR subsets of a given G-ANR space.

2000 Mathematics Subject Classification.   54C55, 55P91.

Key words and phrases.   G-ANR, G-homotopy, G-homotopy extension theorem, slice.

DOI: 10.3336/gm.42.1.05

References:

1. S. A. Antonyan, Retracts in categories of G-spaces, Izv. Akad. Nauk Armyan. SSR Ser. Mat. 15 (1980), 365-378; English translation in: Soviet J. Contemporary Math. Anal. 15 (1980), 30-43.
   MathSciNet

2. S. A. Antonian, Equivariant embeddings into G-ARs, Glas. Mat. Ser. III 22(42) (1987), 503-533.
   MathSciNet

3. S. Antonyan, E. Elfving, and A. Mata-Romero, Adjunction spaces and unions of G-ANE's, Topology Proc. 26 (2001/02), 1-28.
   MathSciNet

4. S. A. Antonyan, Orbit spaces and unions of equivariant absolute neighborhood extensors, Topology Appl. 146/147 (2005) 289-315.
   MathSciNet     CrossRef

5. S. A. Antonyan, Orbit spaces of proper equivariant absolute extensors, Topology Appl. 153 (2005), 698-709.
   MathSciNet     CrossRef

6. K. Borsuk, Theory of Retracts, PWN-Polish Scientific Publishers, Warszawa, 1967.
   MathSciNet

7. G. E. Bredon, Introduction to Compact Transformation Groups, Academic Press, New York-London, 1972.
   MathSciNet

8. T. tom Dieck, Transformation Groups, Walter de Gruyter & Co., Berlin, 1987.
   MathSciNet

9. O. Hanner, Some theorems on absolute neighborhood retracts, Ark. Mat. 1 (1951) 389-408.
   MathSciNet     CrossRef

10. S. T. Hu, Theory of Retracts, Wayne State University Press, Detroit, 1965.
    MathSciNet

11. J. Jaworowski, Extension properties of G-maps, in: Proceedings of the International Conference on Geometric Topology (Warsaw, 1978), PWN, Warsaw, 1980, 209-213.
    MathSciNet

12. J. van Mill, Infinite-dimensional Topology. Prerequisites and Introduction, North Holland Publishing Co., Amsterdam-New York-Oxford-Tokyo, 1989.
    MathSciNet

13. R. S. Palais, The classification of G-spaces, Mem. Amer. Math. Soc. 36, Providence, 1960.
    MathSciNet

14. J. de Vries, Topics in the theory of topological transformation groups, in: Topological Structures II. Math. Centre Tracts 116, Math. Centrum, Amsterdam, 1979, 291-304.
    MathSciNet